Aperiodic monotile.

[Boustrophedon]

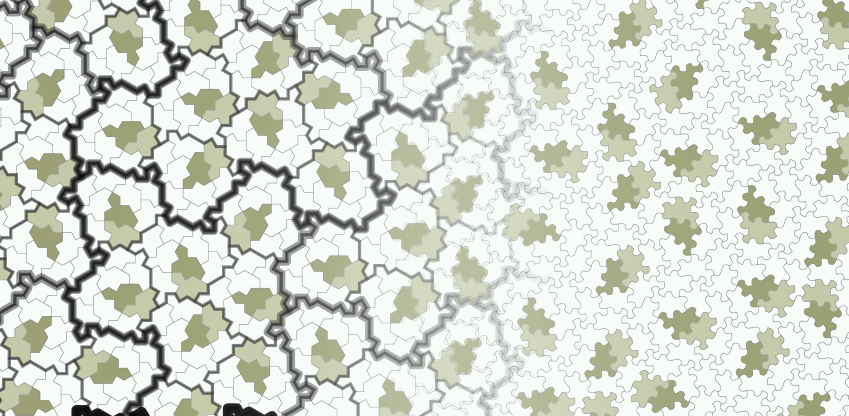
As reported in the New Scientist a group of mathematicians: David Smith, Joseph Samuel Myers,Craig S. Kaplan and Chaim Goodman-Strauss, have claimed to have constructed an aperiodic tiling that uses just one shape of tile.

Paper here: https://arxiv.org/pdf/2303.10798.pdf

This of course beats Penrose tiling which uses two shapes; the dart and the kite.
But to my mind there is just one small niggle, they use the tile and it's reflection. That to my mind is less pure than using congruent tiles without reflection. Certainly spoils it for me. Still it's an important result and really pretty.

1687244959229505550.jpg (91.24 KiB) Viewed 2139 times

[Grumble]

And our very own Jaap hand a hand in it
viewtopic.php?p=145114#p145114

[Boustrophedon]

And our very own Jaap hand a hand in it
viewtopic.php?p=145114#p145114

That's great. I did have a look to see if it had been posted already, didn't find it.

[IvanV]

I can imagine some poor mathematician bashing their head against either trying to prove there cannot be an aperiodic tiling with a single shape of tile (with a finite number of straight sides), counting the reflection as a different tile, or showing the counter-example.

I say finite number of sides, because odd things can happen when you go to the theoretical places beyond that. You could have tilings that change area according to how you arrange the "tiles", as happens in 3-d with the Banach-Tarski paradox.

[Boustrophedon]

And here we go, an aperiodic monotile without reflections.



https://cs.uwaterloo.ca/~csk/spectre/

[IvanV]

And here we go, an aperiodic monotile without reflections.

https://cs.uwaterloo.ca/~csk/spectre/

Amazing. I had not imagined such a quick resolution of that question, given how long it has taken us to get from aperiodic tilings with more than one tile, to aperiodic tiling with one tile. And in fact they have found several - though through fairly simple modifications to the first one.

By drawing attention to the fact that the tile is asymmetric ("chiral"), it means the subject isn't closed yet. It leaves open the question of whether you could have an aperiodic monotile that is symmetric.